this post was submitted on 09 May 2024
1212 points (97.4% liked)
Memes
45727 readers
1034 users here now
Rules:
- Be civil and nice.
- Try not to excessively repost, as a rule of thumb, wait at least 2 months to do it if you have to.
founded 5 years ago
MODERATORS
you are viewing a single comment's thread
view the rest of the comments
view the rest of the comments
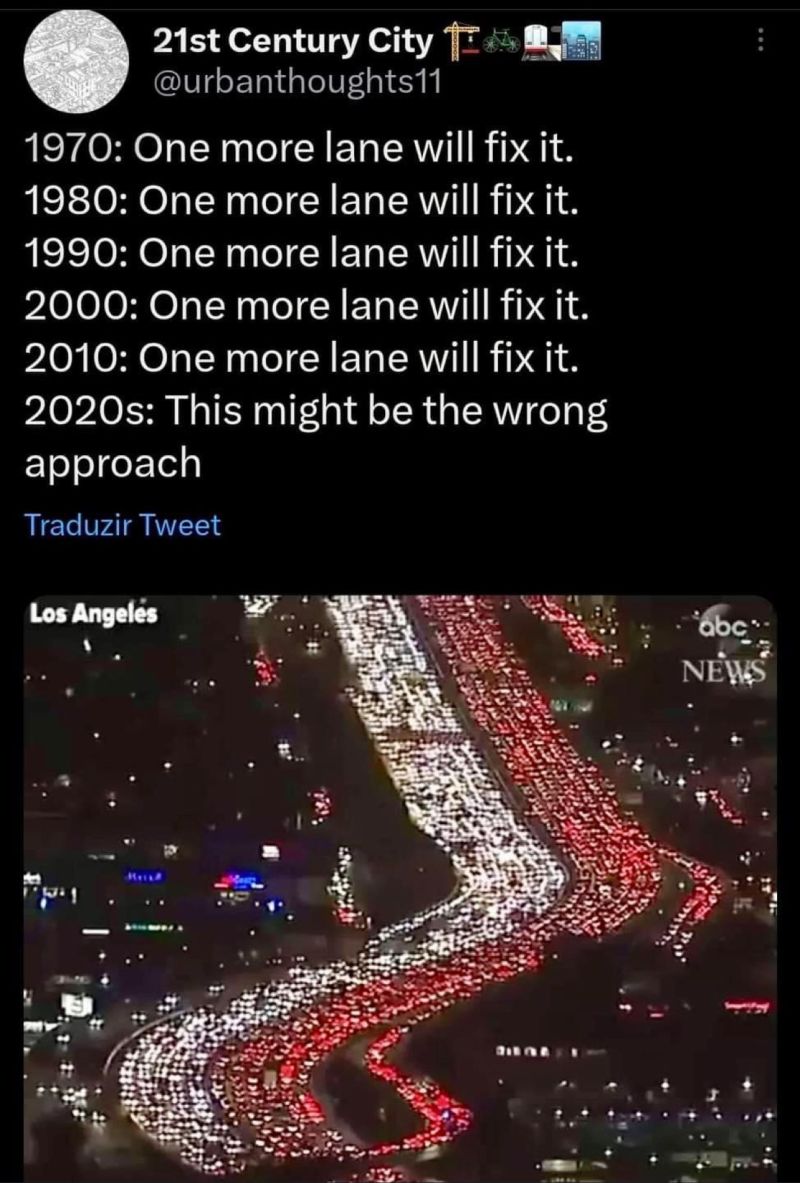
I once heard of an experiment in economics that offers insight into this.
Say you have 100 people. You give each of them one of two choices:
A : you get $40 unconditionally B: you get $70 - n, where n is the number of people who choose B
You end up getting, on average across experiments, n = 30.
If you move the numbers around (i.e, the $40 and the $70), you keep getting, on average, a number of people choosing B so that B pays out the same as A.
I think the interpretation is that people can be categorized by the amount of risk they’re willing to take. If you make B less risky, you’ll get a new category of people. If you make it more risky, you’ll lose categories.
Applied to traffic, opening up a new lane brings in new categories of people who are willing to risk the traffic.
Or something. Sorry I don’t remember it better and am too lazy to look it up. Pretty pretty cool though.
Can you explain what this part means? What do you mean by category here?
Yes. That wasn’t the best word choice; maybe “group” would have been better. I meant groups of people who are willing to take some level of risk. Imagine the categories are “low risk takers”, “medium risk takers”, and “high risk takers”.
Compared to A paying out $40, if you make B $50-n you’ll only get the high risk takers choosing B. If you make it $70-n you’ll get high and medium risk takers. If you make it $120-n you’ll get almost everybody.
If risk taking is a value between 0 and 1, the categories are groups of people inside certain intervals. For example, low could be [0, 1/3), medium could be [1/3, 2/3), and high could be [2/3, 1].