only refers to implicit multiplication involving special expressions(?) like pi, e, sqrt or log, and nothing about “regular” implicit multiplication like 2(1+3)
That was a very astute observation you made there! The fact is, for the very reason you stated, there is in fact no such thing as "implicit multiplication" - it is a term which has been made up by people who have forgotten Terms (the first thing you mentioned) and The Distributive Law (the second thing you mentioned). As you've noted., these are 2 different rules, and lumping them together as one brings exactly the disastrous results you might expect from lumping different 2 rules together as one...
See here for explanation of all the various rules, including textbook references and proofs.
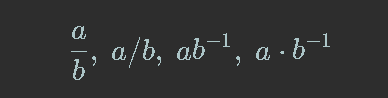



That'd be good, but what I've found so far here is a whole bunch of people who don't like being told the actual facts of the matter! 😂